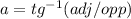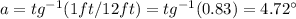Answer:
The maximum angle that a ramp can form with the ground is 4.76°.
Explanation:
Assuming the ramp as a right-angled triangle, and letting the horizontal distance being the leg adjacent and the vertical distance the leg opposite, then the tangent to the ramp angle will be:
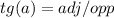
The angle a is solving by the inverse of the leg's rate: