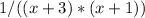Answer:
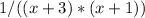
Explanation:
We can write the expression as:
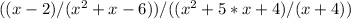
We can write the term as a single division by multiplying the terms in numerator by the inverse on the terms in the denominator:
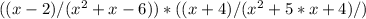

We can simplfy the denominator terms as:
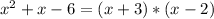
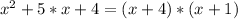
Therefore (x-2) and (x+4) in the numerator and denominator cancels out and we are left with: