Question

Sally is analyzing a circle, y2 + x2 = 9, and a linear function g(x). Will they intersect?

Answer 1

This ones kinda hard I'm not really sure, but looking at the table, when f(x) = 1, g(x) = 1. So therefore it is yes, and Im guessing you know the negative and positive x coordinates/zero thing, so I think you should be correct. Sorry if this is wrong, not too sure, but hopefully it gives you a better idea.

Answer 2

Yes, at negative and positive x-coordinates or zero

Explanation:

we have

`x^(2) +y^(2)=9`

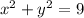
Equation of the circle with center at the origin and radius equal to
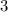`3` units

g(x) -------> a linear function (see the table)

using a graphing tool

Plot the graph of the circle and the three points of the linear function g(x)

Both graphs intersect at negative and positive x-coordinates

see the attached figure