Question

The area of a rectangle is (27x5 + 9x4 - 18x3) square feet. The width of the rectangle is 9x2 feet. What is the length of the rectangle?

DO NOT INCLUDE PARENTHESIS IN YOUR ANSWER

Answer 1

If the width is 9x², then the length is 27x^5+9x^4-18x^3/9x², which equals 3x³+x²+2x. ☺☺☺☺

Answer 2

The length of the rectangle is given by 3x^3+x^2-2x

Explanation:

We have been given that

area of rectangle is
`A=27x^5 + 9x^4 - 18x^3`

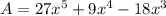
The width of the rectangle is
`w=9x^2`

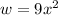
We know the area of rectangle is given by

`A=lw\\\\l=(A)/(w)`

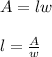
On substituting the known values, we get

`l=(27x^5 + 9x^4 - 18x^3)/(9x^2)`

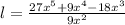
On simplifying this, we get

`l=(27x^5)/(9x^2) +(9x^4)/(9x^2) -(18x^3)/(9x^2) \\\\l=3x^3+x^2-2x`

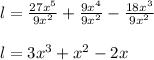
Therefore, the length of the rectangle is given by 3x^3+x^2-2x