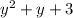Question

The volume of a prism is the product of its height and area of its base, V=Bh. A rectangular prism had a volume of 16y^4 + 16y^3 +48y^2 cubic units. Which could be the base area and height of the prism?

Answer 1

D

Explanation:

Answer 2

Volume = base × height

This is a multiplication relationship, so we need to manipulate the expression
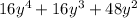
`16y^(4)+ 16y^(3)+ 48y^(2)` into a product of two terms

We achieve this by factorising.
The highest common factor of the constant 16, 16, and 48 is 16
The highest common factor of the variable
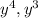
`y^(4), y^(3)`, and
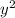
`y^(2)` is
`y^(2)`

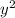
So, factorising
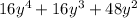
`16y^(4)+ 16y^(3)+ 48y^(2)` gives

`16 y^(2)( y^(2)+ y+3)`

Since the base of the square-based pyramid is a square, the expression of the base would be
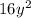
`16y^(2)` (since this expression can be square rooted completely)

The height of the prism will be
`y^(2)+y+3`