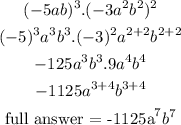Question

What was his mistake and what is the correct answer?

Answer 1

Henry's mistakes were on line 3:

1. He wrote that the square of -3 is -6. When in fact, it should have been 9

2. He did not add the powers of "a" properly. Had He done it properly, He would have gotten 3 + 4 = 7 as the power of "a"

Thus, after correcting his errors, His solution ought to be:

`\begin{gathered} (-5ab)^3.(-3a^2b^2)^2 \\ (-5)^3a^3b^3\text{.}(-3)^2a^(2+2)b^{2+_{}2}_{} \\ -125a^3b^3.9a^4b^4 \\ -1125a^(3+4)b^(3+4) \\ \text{full answer = -1125a}^7b^7 \end{gathered}`