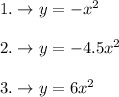Question

Order the group of quadratic functions from widest to narrowest graph. y=6x^2

y=-4.5x^2

y=-x^2

Answer 1

stretches are by the number in front of the x^2 hence the widest is least stretched (-x^2), then -4.5x^2 and lastly 6x^2

Answer 2

Answer with explanation:

The given three Quadratic function are

1. ⇒ y=6 x²

`x^2=(y)/(6)`

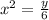
2. ⇒y = -4.5 x²

`x^2=(-y)/(4.5)`

3.⇒y = -x²

`x^2=(-y)/(1)`

In curve 1,Curve 2, and Curve 3, the stretch factor are ,
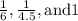`(1)/(6),(1)/(4.5), \text{and} 1` respectively.

`(1)/(6)<(1)/(4.5)< 1`

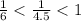
All the three function represent the curve parabola, having vertex at the origin(0,0).

So, order of quadratic functions from widest to narrowest is:

`1.\rightarrow y= -x^2\\\\2.\rightarrow y = -4.5 x^2\\\\3.\rightarrow y=6 x^2`