Question

I NEED HELP RNNNNNNNNNN!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

What is the simplified form of the square root of 64x16 ?

8x4
8x8
32x4
32x8

Answer 1

Option B is correct

`8x^8`

Explanation:

Using the exponent rules:

`\sqrt[n]{a^n} = a`

`\sqrt[n]{a^m} = a^{(m)/(n)}`

Given the expression:

`64x^(16)`

We have to find the simplified form of the square root of the given expression.

Square root of [1] is:

`\sqrt{64x^(16)}`

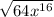
We can write 64 as:

`64 = 8 \cdot 8 = 8^2`

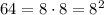
then;

`\sqrt{8^2 \cdot x^(16)}`

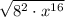
Apply the exponent rules:

`8 \cdot x^{(16)/(2)} = 8 \cdot x^8`

⇒
`8x^8`

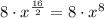
Therefore, the simplified form of the square root of
`64x^(16)` is,
`8x^8`

Answer 2

Option B.
`8x^(8)`

Explanation:

The given expression is
`\sqrt{64x^(16)} =\sqrt{8^(2).x^(8).x^(8)}=\sqrt{8^(2)}.\sqrt{x^(8)}.\sqrt{x^(8)}=8x^(4).x^(4)=8x^(4+4)=8x^(8)`

Therefore the simplified form of the expression will be
`8x^(8)`