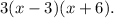Question

Factor completely 3x2 + 9x − 54.l

Answer 1

3x^2 + 9x - 54
3(x^2 + 3x - 18)
3(x + 6)(x - 3)

Answer 2

Answer: The complete factorization of the given expression is
`3(x-3)(x+6).`

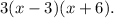
Step-by-step explanation: We are given to factor completely the following quadratic expression :

`E=3x^2+9x-54\\\\\Rightarrow E=3(x^2+3x-18)~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

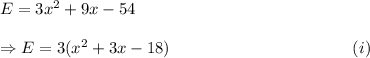
To completely factor expression (i), we need two integers with sum 3 and product -18. Those two integers are 6 and -3.

The complete factorization of expression (i) is as follows :

`E\\\\=3(x^2+3x-18)\\\\=3(x^2+6x-3x-180)\\\\=3(x(x+6)-3(x+6))\\\\=3(x-3)(x+6).`

Thus, the complete factorization of the given expression is
`3(x-3)(x+6).`