Question

Which expression is equivalent to the following complex fraction?

(1-1/x)/2
A) x-1/2x
B) -1/2
C) 2x-2/x
D) 2x/x-1

Answer 1

A)
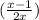`((x - 1)/(2x))` is equivalent fraction.

Step-by-step explanation:

Given :
`(1-(1)/(x))/(2)`.

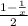To find : Which expression is equivalent to the following complex fraction.

Solution : We have given that
`(1-(1)/(x))/(2)`.

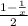Rewrite the equation
`(1-(1)/(x))/(2)`=
`(1 - (1)/(x) )*(1)/(2)`.

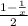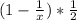Taking x as LCD in numerator

`(1-(1)/(x))/(2)` =
`((x - 1)/(x))*(1)/(2)`

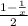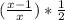
On multiplying denominator x and 2

`(1-(1)/(x))/(2)` =
`((x - 1)/(2x))`.

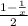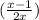Therefore, A)
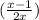`((x - 1)/(2x))` is equivalent fraction.

Answer 2

Answer: The correct option is A.

Step-by-step explanation:

The given expression,

`\frac{1-(1)/(x)} {2}`

First we have to simplify the numerator terms.

`\frac{1-(1)/(x)} {2}=(1-(1)/(x))*(1)/(2)`

Take x as LCD in numerator.

`\frac{1-(1)/(x)} {2}=((x-1)/(x))*(1)/(2)`

It can be written as,

`\frac{1-(1)/(x)} {2}=(x-1)/(2x)`

Therefore the given complex fraction can be expressed as
`(x-1)/(2x)`

This expression is same as the expression written in option A. So, the option A is correct.