Question

A jar contains 8 nickels, 10 dimes, 12 quarters, and 22 pennies. a coin is chosen at random from the jar. what is the probability that the coin chosen is a penny? question 11 options:

Answer 1

22/(22+10+8+12)=22/52=11/26

Answer 2

Answer: The required probability is 42.30%.

Step-by-step explanation: We are given that a jar contains 8 nickels, 10 dimes, 12 quarters, and 22 pennies. a coin is chosen at random from the jar.

We are to find the probability that the coin chosen is a penny.

Let S denotes the sample space for experiment of selecting a coin of the jar and A denotes the event that the randomly selected coin is a penny.

Then,

n(S) = 8 + 10 + 12 + 22 = 52 and n(A) = 22.

Therefore, the probability of event A is given by

`P(A)=(n(A))/(n(S))=(22)/(52)=(11)/(26)* 100\%=42.30\%.`

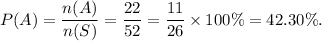
Thus, the required probability is 42.30%.