Question

If the base-dissociation constant, Kb, for a substance is 4.3 x 10^–10, what does this tell you about Ka?

Answer 1

Ka*Kb=Kw=10^-14
Ka=10^-14/(4.3*10^-10) (at 25°C)

Answer 2

Answer: The acid dissociation constant for the given substance is
`2.32* 10^(-5)`

Step-by-step explanation:

To calculate the acid dissociation constant for the given base dissociation constant, we use the equation:

`K_w=K_b* K_a`

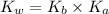
where,

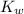`K_w` = Ionic product of water =
`10^(-14)`

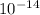
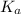`K_a` = Acid dissociation constant

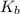`K_b` Base dissociation constant =
`4.3* 10^(-10)`

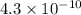
Putting values in above equation, we get:

`10^(-14)=4.3* 10^(-10)* K_a\\\\K_a=(10^(-14))/(4.3* 10^(-10))=0.232* 10^(-4)=2.32* 10^(-5)`

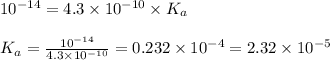
Hence, the acid dissociation constant for the given substance is
`2.32* 10^(-5)`