Answer:
The correct option is D. 3 is an outlier.
Explanation:
A data set has outliers if the data lie out side the interval
![I=[Q_1-1.5* IQR,Q_3+1.5* IQR]](https://img.qammunity.org/2018/formulas/mathematics/high-school/e1wa8l066cqgd8qlpzuqn75y6j7msqwmow.png)
The interquartile range of a data set is
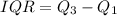
For option A, the required interval is [5.5, 25.5]. Since all the data lie in this interval, therefore this data set has no outliers.
For option B, the required interval is [-5, 19]. Since all the data lie in this interval, therefore this data set has no outliers.
For option C, the required interval is [-2.5, 17.5]. Since all the data lie in this interval, therefore this data set has no outliers.
For option D,
3, 6, 7, 7, 8, 8, 9, 9, 9, 10
(3, 6), 7, (7, 8),( 8, 9), 9,( 9, 10)
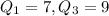
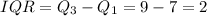
![I=[Q_1-1.5* IQR,Q_3+1.5* IQR]](https://img.qammunity.org/2018/formulas/mathematics/high-school/e1wa8l066cqgd8qlpzuqn75y6j7msqwmow.png)
![I=[7-1.5* 2,9+1.5* 2]](https://img.qammunity.org/2018/formulas/mathematics/high-school/o7pp3i5vh3ugrsblut0bn7c09xz2bgcqid.png)
![I=[7-3,9+3]](https://img.qammunity.org/2018/formulas/mathematics/high-school/ihksog2h2vxem0npqrn2qrfneapwh5b2i6.png)
![I=[4,12]](https://img.qammunity.org/2018/formulas/mathematics/high-school/jb5qjsh7njt8t3bdut0teljio5gyyrce9e.png)
3 lies out side the interval [4,12]. It means this data set has an outlier, i.e,.
Therefore correct option is D.