Answer:
Option (C) is correct.
Thus, the result of
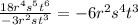
Explanation:
Given: expression

We have to find the value of given expression and choose a correct option from the given options.
Consider the given expression

Apply fraction rule,
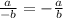
We have,

Divide 18 by 3 , we get 6,
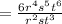
Apply exponent rule
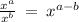
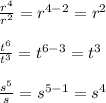
we get,

Thus, the result of
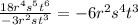
Option (C) is correct.