ANSWER :
0.3859
EXPLANATION :
The z-score formula is :
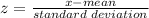
The mean is 71 inches and the standard deviation is 3.5 inches
Calculate z when x = 6 feet or 72 inches
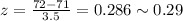
Using the normal distribution table :
0.6141 is the area under the curve or the percentage of adult men that are below 6 feet, since the area of the curve is to the left of z.
We need the area under the curve which is to the right of z.
Recall that the total area under the curve is 1.
Then we need to subtract 0.6141 from 1.
1 - 0.6141 = 0.3859