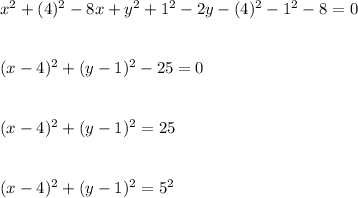Question

The equation for a circle is ​x2−8x+y2−2y−8=0​ .

What is the equation of the circle in standard form?

Answer 1

the eq of the circle in standard form is x^2+y^2+2ux+2vy +d=0 where (-u,-v) is the centre and sq root of u^2+v^2-d is the radius

Answer 2

The equation of the given circle in standard form is:

`(x-4)^2+(y-1)^2=5^2`

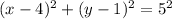
Explanation:

The standard form of a circle is given by the equation:

`(x-h)^2+(y-k)^2=r^2`

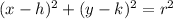
where (h,k) represent the center of the circle and r denotes the radius of the circle.

Hence, we are given equation as:

`x^2-8x+y^2-2y-8=0`

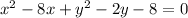
On solving as follows:

`x^2+(4)^2-8x+y^2+1^2-2y-(4)^2-1^2-8=0\\\\\\(x-4)^2+(y-1)^2-25=0\\\\\\(x-4)^2+(y-1)^2=25\\\\\\(x-4)^2+(y-1)^2=5^2`