Question

Domain of two functions

Answer 1

`\bf f(x)=x+7\qquad g(x)=\cfrac{1}{x-13} \\\\\\ (f\circ g)(x)\iff f[\quad g(x)\quad ]=[g(x)]+7 \\\\\\ f[\quad g(x)\quad ]=\cfrac{1}{x-13}+7\implies f[\quad g(x)\quad ]=\cfrac{1+7(x-13)}{x-13} \\\\\\ f[\quad g(x)\quad ]=\cfrac{1+7x-91}{x-13}\implies f[\quad g(x)\quad ]=\cfrac{7x-90}{x-13}`

domain are the values "x" can safely take, without yielding an unworkable expression

for fractions an unworkable one, happens if the denominator is 0, if the denominator of a fraction is 0, then the fraction becomes undefined

now, the denominator there is still x-13, what values of "x" makes it undefined? or 0, well, let's check

x - 13 = 0
x = 13

so if "x" ever becomes 13, the denominator turns to 13 -13, which is zero, giving us
`\bf \cfrac{7x-90}{0}\impliedby \textit{und}\textit{efined}`

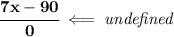
so.. the domain is all values EXCEPT 13, those "x" can safely take

x