ANSWER :
f(x) = (3x-2)(x-3)(x-4)(x-2)^3
EXPLANATION :
A polynomial with a degree of 6 has 6 factors.
f(x) = (x - a)(x - b)(x - c)(x - d)(x - e)(x - f) with 6 roots or x-intercepts.
But the problems states that it has 4 x-intercepts, so we will reduced the number of roots but maintaining the number of factors.
f(x) = (x - a)(x - b)(x - c)(x - d)(x - a)(x - a).
From here, we still have 6 factors but only 4 x-intercepts, the last two factors (x - a) is the same as the first factor.
So we can rewrite this as :
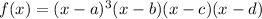
Next is to have a y-intercept of 64, y-intercept is the value of f(x) when x = 0
Substitute 0 to the function.
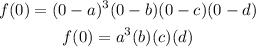
Now we have f(0) = a^3bcd and f(0) = 64 as the definition from above.
We need to find the factors of 64,
64 = 8 x 4 x 3 x 2/3
And we can rewrite the equation as :
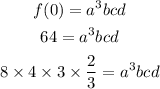
From here, we can observe that,
a^3 = 8 ⇒ a = 2
b = 4
c = 3
d = 2/3
So the function will be :

Explanation in 2/3
Since we only need 4 distinct factors of 64.
8 x 4 x 3 x 2/3
8 x 4 = 32
The product of the 3rd and 4th factor should be 2, in order to get 64.
Since from the first