Answer: 81.33 square units
Explanation:
Given sides of triangle = 12,14 and 16 are the a,b and c respectively.
Thus the perimeter of triangle
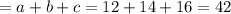
The semi perimeter of triangle
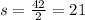
By Heron's formula
Area of triangle =
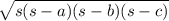
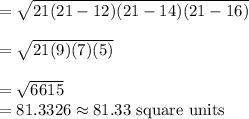
The area of triangle =81.33 square units.