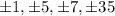Question

A new company estimates its total profit (profit = total revenue minus total cost) as P(x) = x4 – 2x3 – 240x – 35, where P is in hundreds of dollars and x is the number of months elapsed since the company’s start-up. According to the rational zero theorem, what can be the values of x until the company breaks even?

Answer 1

With ruffini you easily get 5.6666 months

Answer 2

Answer:
`\pm 1,\pm5,\pm7,\pm35`

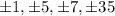
Explanation:

Given: A new company estimates its total profit as

`P(x) = x^4 - 2x^3 - 240x - 35`, where P is in hundreds of dollars and x is the number of months elapsed since the company’s start-up.

The coefficient of the leading term (a)= 1

The constant term = 35

The factors of 35 (b)=
`\pm 1,\pm5,\pm7,\pm35`

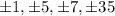
By rational root theorem , we have

The rational zeros
`(b)/(a)=(\pm 1)/( 1),(\pm 5)/(1),(\pm7)/(1),(\pm35)/(1)`

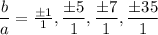
Hence, the values of x until the company breaks even =
`\pm 1,\pm5,\pm7,\pm35`