Question

Help giving medals find the area of the triangle with the given measurements. round the solution to the nearest hundredth if necessary. b = 104°, a = 11 cm, c = 18 cm 192.12 cm2 96.06 cm2 23.95 cm2 99 cm2

Answer 1

In order to solve for the area of the triangle with the given dimensions, we use the equation

A = ac(cos b)/2

Substituting the known values,

A = (11 cm)(18 cm)(cos 104o)/2 = 23.95 cm2

Thus, the answer is the third choice.

Answer 2

Option B is correct.

Explanation:

Given: In a ΔABC, ∠B = 104° , a = 11 cm and c = 18 cm.

To find: area of the triangle.

We first find value of b using Law of cosines then using herons formula we find area of triangle.

Law of Cosines is a result used for calculating one side of a triangle when the angle opposite and the other two sides are known.

b² = a² + c² - 2ac × cos B

b² = 11² + 18² - 2 × 11 × 18 × cos 104°

b² = 445 - 396 × ( -0.24 )

b² = 540.04

b = 23.24 (nearest tenth)

Now, Herons Formula,

Semi perimeter,
`s=(a+b+c)/(2)=(11+23.24+18)/(2)=(52.24)/(2)=26.12`

`Area=√(s(s-a)(s-b)(s-c))=√(26.12(26.12-11)(26.12-23.24)(26.12-18))`

`=√(26.12*15.12*2.88*8.12)=√(9235.78)=96.02`

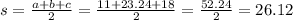
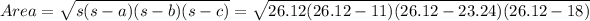
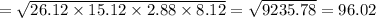
Area of the triangle = 96.02 cm²

Therefore, Option B is correct.