Question

write the particular equation of this transformed sine graph. assume that the horizontal shift is 1 unit to the right. (hint: try to find the best point to center the sinusoidal axis.)

Answer 1

We are given the graph of sine function.
First, we get the amplitude
A = [6 - (-2)] / 2
A = 4

Next, we determine the period and b
T = 4 - 0 = 4
b = 2π / T
b = π/2

The original sine function was
y = 4 sin πx/2

After the transformation, the equation now is
y = 4 sin [π(x+2)/2] + 2

Answer 2

READ THE QUESTION CAREFULLY.

y=4sin(
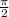`(\pi )/(2)`(x-1))+2

Explanation:

Amplitude: Max is at 6, Min is at -2. Halfway point is 2. Subtract Max and Min with Halfway point.

6-2=4

2-(-2)=4

Thus Amplitude is 4.

The Max is at 6. The Min is at -2. Halfway point is at 2. Draw a horizontal line. That is your D. Since the graph has a horizontal shift 1 unit to the right, that will be your C. Draw a vertical line on 1. Usually Sinusoidal axis are (C,D).

Thus (C,D) = (1,2)

Then you need to find the period. When does one mountain completely form? The Period is 4. (Confused? Draw a mountain and see how it starts and ends).

Thus 4=
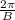`(2\pi )/(B)`⇒B=
`(2\pi )/(4)`⇒
`(\pi )/(2)`

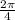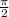
Then put it all together.

General Form: Asin(B(x-C))+D

A=4 , B=
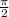`(\pi )/(2)` , C= 1 , D=2

Answer:

y=4sin(
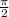`(\pi )/(2)`(x-1))+2