Question

A building has a ramp to its front doors to accommodate the handicapped. if the distance from the building to the end of the ramp is 22 feet and the height from the ground to the front doors is 6 feet, how long is the ramp? (round to the nearest tenth.)

Answer 1

Answer:The length of the ramp is 22.8 feet.

Step-by-step explanation:

The distance of the ramp end from the building = AB = 22 feet

Height from the ground to front door = BC = 6 feet

The length of the ramp AC.

According to Pythagoras theorem.

`AC^2=AB^2+BC^2`

`AC=√(22^2+6^2)=22.8 feet`

The length of the ramp is 22.8 feet.

Answer 2

Answer : Length of the ramp, AC = 22.8 ft.

Explanation :

From the attached figure,

Height of building, AB = 6 ft

Distance from the building to the end of the ramp, BC = 22 ft

Using Pythagoras theorem :

`AC^2=BC^2+AB^2`

`AC=√(BC^2+AB^2)`

`AC=√(22^2+6^2)`

`AC=22.8\ ft`

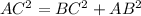
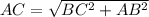
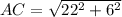
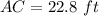
The length of the ramp is 22.8 ft

Hence, this is the required solution.