Question

Part A: Solve –vp + 40 < 65 for v. Show your work. (4 points) Part B: Solve 7w – 3r = 15 for r. Show your work. (6 points)

Answer 1

A.

remember when you multiply or divide by a negative with inequalities, flip the direction of the sign
-vp+40<65
minus 40 both sides
-vp<25
divide both sides by -p and flip sign
v>-25/p






B.
minus 7w from both sides
-3r=15-7w
divide both sides by -3
r=(15-7w)/(-3)
r=(15/-3)+(-7w/-3)
r=-5+(7w)/3

Answer 2

Solve for v,
`v>-(25)/(p)`

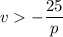
Solve for r,
`r=(7)/(3)w-5`

Explanation:

Part A:

Given:
`-vp+40<65`

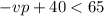
We need to solve for v. We have to isolate v.

`-vp+40<65`

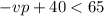
Subtract 40 both sides

`-vp<65-40`

`-vp<25`

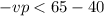
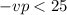
Divide by -p both sides and change the sign of inequality ( because if we divide by negative number inequality change)

`v>-(25)/(p)`

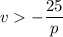
Part B:

Given:
`7w-3r=15`

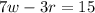
We need to solve for r. We have to isolate r.

`7w-3r=15`

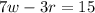
Subtract 7w both sides

`-3r=15-7w`

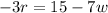
Divide by both sides -3

`r=(15-7w)/(-3)`

`r=(7)/(3)w-5`