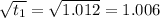1. Relative extrema of functions are the "tops" or the "bottoms" of the curves (the graphs) representing functions. So they are the points at which the tangent lines are horizontal.
2. In short we are looking for the solutions of f'(x)=0
3. f'(x)=
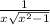
-9=0

Notice:

so that the square root of this expression exists. So x

1 or x
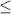
-1
Also in

notice that the root is always positive and 1/9 is positive, so x must be positive. This second condition, combined with the first one tells us that the solution is in x

1
4.

Square both sides
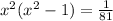
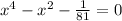
let
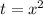
Then the 4th degree equation becomes the quadratic equation
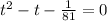
The discriminant D=

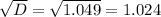


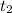
is not considered as
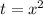
so t cannot be negative
x=