Question

Two golf balls are hit from the same point on a flat field. Both are hit at an angle of 30∘ above the horizontal. Ball 2 has twice the initial speed of ball 1.

If ball 1 lands a distance d1 from the initial point, at what distance d2 does ball 2 land from the initial point? (Neglect any effects due to air resistance.)

Answer 1

First let's find the time it takes for the first ball to land:
Acceleration is a=-g so vertical velocity is V=-gt + V1sin(30).
Position is thus
S=(-1/2)gt^2 +V1t sin(30).
Solving for t gives
t=2V1sin(30)/g
The second ball has the same position function except for the new velocity, which is given by
V2=2V1. Putting this in and solving for t2 gives
t2=4V1sin(30)/g.
It takes twice as long for the second ball to land on the ground.
The horizontal distance of ball 1 is S1 = V1t cos(30). Again we look at ball 2's distance by substituting V2=2V1 and get
S2 = 2V1t2 cos(30).
Note here I put in t2 since it will fly for that amount of time. But we already saw that
t2 = 2t1
So S2=4V1 cos(30)
That is the second ball goes 4 times further than the first one. This is because it is going twice as fast along both the horizontal and the vertical. It moves horizontally twice as fast for twice as long.

Answer 2

the distance of second ball will be 4 times more than the distance of first ball.

Step-by-step explanation:

Since both the balls are hit from ground level and reached again at ground then the horizontal distance covered by the ball must be equal to the range of projectile motion

As we know that range of projectile motion is given as

`R = (v^2 sin(2\theta))/(g)`

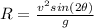
so here we can say that for the first ball is the speed is "v" then its distance on the ground will be

`d_1 = (v^2 sin(2\theta))/(g)`

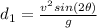
now for the other ball if the speed is double but the angle is same so we have

`d_2 = (4v^2 sin(2\theta))/(g)`

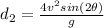
so the distance of second ball will be 4 times more than the distance of first ball.