Question

Help needed;( A woman wants to build a rectangular garden. She plans to use a side of a shed for one side of the garden. She has 84 yards of fencing material.

What is the maximum area that will be enclosed?



Enter your answer in the box.

Answer 1

Let x denote the length of the side of the garden which is covered fenced by a shed, and
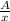
`(A)/(x)` be the width of the garden.

The perimeter of a rectangle is given by 2(length + width).
Given that on of the sides is to be covered by the side of a shed, the the perimeter of the remaining three sides to be fenced is given by

`x+ 2\left( (A)/(x) \right)=84`
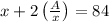
which gives:

`2A=84x-x^2 \\ A=42x- (1)/(2) x^2`
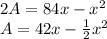
For the area to be maximum, the differentiation of A with respect to x must be equal to 0.
i.e.
`(dA)/(dx) =42-x=0 \\ x=42`
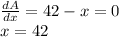
Therefore, the maximum area of the garden enclosed is given by

`A_(max)=42(42)- (1)/(2) (42)^2= (1)/(2) (1764)=882 \, yd^2.`