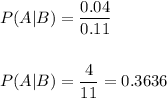2 Answers

Martin Fink · Answer 1 · 2018-01-14T12:06:36+0000

Answer:

The probability is:

(4)/(11)

Explanation:

It is given that:

16% of the student population is taking a calculus course.

11% is taking a physics course.

and 4% is taking both.

Now we are asked to find the probability that the student is taking a calculus course given that he is taking physics course.

Let A denote the event of taking a calculus course.

B denote the event of taking a physics course.

A∩B denote the event of taking both.

Let P denote the probability of an event.

We are asked to find:

P(A|B)

We know that:

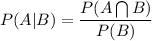
$P(A|B)=(P(A\bigcap B))/(P(B))$

From the information we have:

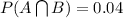
$P(A\bigcap B)=0.04$

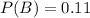
P(B)=0.11

Hence,

$P(A|B)=(0.04)/(0.11)\\\\\\P(A|B)=(4)/(11)=0.3636$

Please log in or register to add a comment.