Question

A triangle has side lengths of 18 cm , 80 cm, and 81 cm. Is this triangle Obtuse Acute or Right. And please explain how you got your answer so i can check it

Answer 1

acute because its not 90 degrees and it isnt greater then 90

Answer 2

The triangle is acute.

Explanation:

Given : A triangle has side lengths of 18 cm , 80 cm, and 81 cm.

To find : Is this triangle Obtuse Acute or Right.

Solution :

According to property of triangle,

If the square of larger side of triangle is equating to the sum of square of smaller side

If
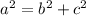`a^2=b^2+c^2` the triangle is right triangle

If
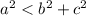`a^2<b^2+c^2` the triangle is acute triangle

If
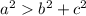`a^2>b^2+c^2` the triangle is obtuse triangle

where, a is the larger side and b,c are the smaller side

Let a=81 , b=80, c=18

LHS-

`a^2=81^2=6561`

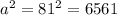
RHS -

`b^2+c^2=80^2+18^2\\b^2+c^2=6400+324=6724`

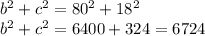
LHS <RHS

Means
`a^2<b^2+c^2`

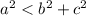
Therefore, The triangle is acute.