Question

By using the equation below complete the square, write the equation of the circle in standard form from the following equation. Then determine the center and radius of

a circle.

10y + x2 = -18+ 5x - y2

Answer 1

Given:

The equation of a circle is

`10y+x^2=-18+5x-y^2`

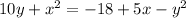
To find:

The center and radius of the given equation by completing the square.

Solution:

The standard form of a circle is

`(x-h)^2+(y-k)^2=r^2` ...(i)

where, (h,k) is center and r is radius of the circle.

We have,

`10y+x^2=-18+5x-y^2`

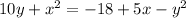
It can be written as

`(x^2-5x)+(y^2+10y)=-18`

`\left(x^2-5x+\left((5)/(2)\right)^2\right)+\left(y^2+10y+\left((10)/(2)\right)^2\right)=-18+\left((5)/(2)\right)^2+\left((10)/(2)\right)^2`

`\left(x-(5)/(2)\right)^2+\left(y^2+10y+5^2\right)=-18+(25)/(4)+5^2`

`\left(x-(5)/(2)\right)^2+(y+5)^2=-18+(25)/(4)+25`

`\left(x-(5)/(2)\right)^2+(y+5)^2=(-72+25+100)/(4)`

`\left(x-(5)/(2)\right)^2+(y+5)^2=(53)/(4)`

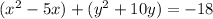
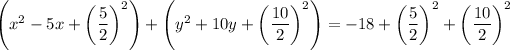
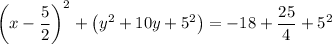
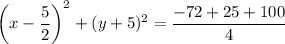
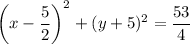
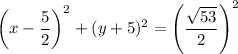`\left(x-(5)/(2)\right)^2+(y+5)^2=\left((√(53))/(2)\right)^2` ...(ii)

On comparing (i) and (ii), we get

`h=(5)/(2),k=-5,r=(√(53))/(2)`

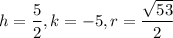
Therefore, the center is
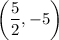`\left((5)/(2),-5\right)` and the radius is
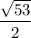`(√(53))/(2)` units.