Question

If the amplitude of a large quake is 1,000 times greater than the amplitude of a smaller quake, what is the difference in their magnitudes on the Richter scale? Use the following formula to find the difference, where ML and MS represent the magnitudes of the larger and smaller quakes, respectively, and AL and AS represent the amplitudes of the larger and smaller quakes, respectively.

The formula is M(l)-M(s)=log(A(l)/A(s))

Answer 1

The difference between the magnitudes of the earthquakes is 3.

Explanation:

We are given that,

The amplitude of large earthquake is 1000 times the amplitude of small earthquake.

That is,
`A(L)=1000* A(S)`

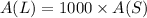
It is required to find the difference between magnitudes of the larger and smaller earthquakes.

We have the formula,

`M(L)-M(S)=\log {(A(L))/(A(S))`

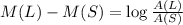
i.e.
`M(L)-M(S)=\log {(1000* A(S))/(A(S))`

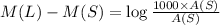
i.e.
`M(L)-M(S)=\log 1000`

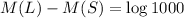
i.e.
`M(L)-M(S)=3`

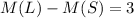
Thus, the difference between the magnitudes of the earthquakes is 3.

Answer 2

So we want to know what is the difference in magnitude of a large quake M(l) and a small quake M(s) in the Richter scale if the amplitude of a large quake A(l) is 1000 times bigger than the amplitude of a small quake A(s) and the formula is M(l)-M(s)=log{A(l)/A(s)}. So the large amplitude is 1000 bigger than the small amplitude: A(l)=1000*A(s). When we insert that into the formula we get:

M(l)-M(s)=log{1000*A(s) / A(s)}, because the A(s) cancel out, so we get:
M(l)-M(s) = log{1000} = 3.

So the difference in magnitude on the Richter scale is M(l)-M(s)=3.