Question

Finding a different quotient for a linear or quadratic function

Answer 1

We need to calculate the expression for f(x+h) before we can find the difference quotioent.

`\begin{gathered} f(x+h)=5\cdot(x+h)^2+1 \\ f(x+h)=5x^2+10hx+5h^2+1 \end{gathered}`

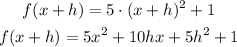
Now we can replace the value of f(x+h) and the value of f(x) on the expression, and simplify it as much as possible to determine the quotient.

`\begin{gathered} (5x^2+10hx+5h^2+1-5x^2-1)/(h) \\ (10hx+5h^2)/(h) \\ (h(10x+5h))/(h) \\ 10x+5h \end{gathered}`

The value of the quotient is "10x + 5h"