Question

The triangle PQR shown on the coordinate grid below is reflected once to map onto triangle P'Q'R': Triangle PQR drawn on a 4 quadrant coordinate grid with vertices P is at 7, 6. R is at 5, 2. Q is at 3, 5. If vertex P' is at (7, −6), what are the coordinates of vertex R'? (5, −3) (3, −5) (3, −2) (5, −2)

Answer 1

The correct option is 4. The coordinates of R' is (5,-2).

Explanation:

It is given that after reflection the triangle P'Q'R' is an image of triangle PQR.

If the figure reflects across the x-axis then the x coordinate remains same but the sign of y-coordinate change.

`(x,y)\rightarrow (x,-y)`

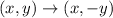
If the figure reflects across the y-axis then the y coordinate remains same but the sign of x-coordinate change.

`(x,y)\rightarrow (-x,y)`

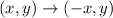
It is given that P(7,6) and P'(7,-6). Since the x coordinate remains same, therefore the figure PQR reflects across the x-axis.

The point is Q(3,5).

`(3,5)\rightarrow (3,-5)`

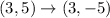
The point is R(5,2).

`(5,2)\rightarrow (5,-2)`

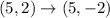
Therefore the coordinates of R' is (5,-2). Option 4 is correct.

Answer 2

If we notice points P and P', the transformation preserves every point along x direction and moves the point by twice its y-coordinate down along y direction.

So, point P moved from (7, 6) to (7, 6-12) = (7, -6).

Hence, point R (5, 2) would be moved to (5, 2-4) = (5, -2).