Answer:
d. 0.26
Explanation:
We are asked to find the value of the The sine of the complimentary angle to 75°.
Since we know that complementary angles add up-to 90 degrees, so let us find the measure of complementary angle of 75° angle by subtracting 75° from 90°.
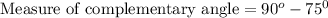
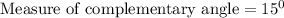
Now let us find the sine of 15 degree angle. Using a calculator we will get,
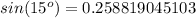
Upon rounding our answer to nearest hundredth we will get,
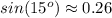
Therefore, the sine of complementary angle to 75° is 0.26 and option 'd' is the correct choice.