Answer:
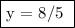
Step-by-step explanation:
[to solve for y, first use the properties of equality to simplify the equation]
5y + 3 = 8y − 5 + 2y
5y + 3 = (8y + 2y) – 5
[regroup the like terms of y together: commutative property of equality ; adding in a different order will still give you the same result]
5y + 3 = 10y – 5
[combine like terms]
5y + 3 = 10y – 5

[subtract 3 from both sides in order to eliminate the constant term on the left side: subtraction property of equality]
5y = 10y – 8
–10y –10y
[subtract 10 from both sides in order to eliminate the variable term: subtraction property of equality]
-5y = -8
÷(-5) ÷(-5)
[divide both sides by -5 to cancel out the coefficient of y: division property of equality]
y = 8/5