Question

Find the values of x and y when the smaller triangle has an area of 54 cm2.The value of x is cm and the value of y is cm.(Type exact answers, using radicals as needed. Rationalize all denominators.)

Answer 1

The two triangles are similar triangles. As such, the following is true:

`(\frac{\text{side length of smaller triangle}}{\text{side length of bigger triangle}})^2=\frac{Area\text{ of smaller triangle}}{\text{Area of bigger triangle}}`

Thus, we first have to compute the area of the bigger triangle, as follows:

`\text{Area =}(1)/(2)* base* height`

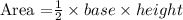
Since, for the bigger triangle, base = 81cm, and height = 36cm, we have:

`\begin{gathered} \text{Area =}(1)/(2)* base* height \\ \Rightarrow\text{Area =}(1)/(2)*81*36 \\ \Rightarrow\text{Area =}(2916)/(2)=1458 \\ \Rightarrow Area=1458cm^2 \end{gathered}`

Now, we find x and y, as follows:

`\begin{gathered} ((x)/(36))^2=(54)/(1458) \\ \Rightarrow((x)/(36))^2=(1)/(27) \\ \Rightarrow(x)/(36)=\sqrt[]{(1)/(27)}=\frac{1}{\sqrt[]{27}} \\ \Rightarrow(x)/(36)=\frac{1}{\sqrt[]{9*3}}=\frac{1}{\sqrt[]{9}*\sqrt[]{3}}=\frac{1}{3*\sqrt[]{3}} \\ \Rightarrow x=36*\frac{1}{3*\sqrt[]{3}}=\frac{36}{3\sqrt[]{3}}=\frac{12}{\sqrt[]{3}} \\ \Rightarrow x=\frac{12}{\sqrt[]{3}}*\frac{\sqrt[]{3}}{\sqrt[]{3}}=\frac{12\sqrt[]{3}}{\sqrt[]{9}}=\frac{12\sqrt[]{3}}{3}=4\sqrt[]{3} \\ \Rightarrow x=4\sqrt[]{3}\text{ cm} \end{gathered}`

Now, y can be obtained similarly:

`\begin{gathered} ((y)/(81))^2=(54)/(1458) \\ \Rightarrow((y)/(81))^2=(1)/(27) \\ \Rightarrow(y)/(81)=\sqrt[]{(1)/(27)}=\frac{1}{\sqrt[]{27}} \\ \Rightarrow(y)/(81)=\frac{1}{\sqrt[]{9*3}}=\frac{1}{\sqrt[]{9}*\sqrt[]{3}}=\frac{1}{3*\sqrt[]{3}} \\ \Rightarrow y=81*\frac{1}{3*\sqrt[]{3}}=\frac{81}{3\sqrt[]{3}}=\frac{27}{\sqrt[]{3}} \\ \Rightarrow y=\frac{27}{\sqrt[]{3}}*\frac{\sqrt[]{3}}{\sqrt[]{3}}=\frac{27\sqrt[]{3}}{\sqrt[]{9}}=\frac{27\sqrt[]{3}}{3}=9\sqrt[]{3} \\ \Rightarrow y=9\sqrt[]{3}\text{ cm} \end{gathered}`