Question

A vehicle travels a distance of 5t^4 - 10t^2+6 miles in t+2 minutes. the vehicles speed is

Answer 1

`s=5t^(3)-10t^(2)-10t-20+(46)/(t+2)`

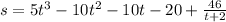
Explanation:

To find answer, we have to use the relation of the movement

`d=st`

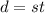
Where, the speed would be

`s=(d)/(t)`

Now, the given expressions are about distance and time, if we replace this expression in the formula above, we would have to divide both polynomials as follows

`s=(5t^(4)-10t^(2)+6)/(t+2)`

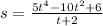
If we divide, we would have a long division as it's attached. And the result would be

`s=5t^(3)-10t^(2)-10t-20+(46)/(t+2)`

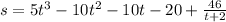
Because according to Euclid's algorithm:

`(D)/(d)=q+r`

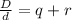
So, the fraction at the end, it's because the division is not exact, that is, the reminder is different than zero.

Answer 2

Speed is calculated as distance over time. S = D/t, given the distance of 5t^4 - 10t^2+6 miles and time is t+2 minutes, we will use the method of division of polynomials. The speed is 5t^3 - 10t^2 + 10t - 20 + 46/(t+2) miles per minute