Question

Determine the open intervals on which the function is increasing, decreasing, or constant. (Enter your answers using interval notation.

Answer 1

• Increasing: (4, ∞)

,

• Decreasing: (-∞. 4)

,

• Constant: DNE

Explanation:

Given the function:

`f(x)=x^2-8x`

First, find the derivative:

`f^(\prime)(x)=2x-8`

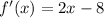
When f'(x)<0:

`\begin{gathered} 2x-8<0 \\ 2x<8 \\ x<(8)/(2) \\ x<4 \\ \implies(-\infty,4) \end{gathered}`

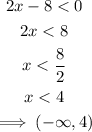
The interval of decrease is at (-∞, 4).

When f'(x)>0:

`\begin{gathered} 2x-8>0 \\ 2x>8 \\ x>(8)/(2) \\ x>4 \\ \implies(4,\infty) \end{gathered}`

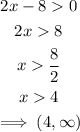
The interval of increase is at (4, ∞).

There is no interval at which the function is constant, so we write DNE.