Answer: 120
Explanation:
The given letters : P Q R S T
Number of letters : 5
The formula of number of permutations of n things taken r at a time is given by :-

Similarly, the number of permutations of 5 letters taken four at a time is given by :-
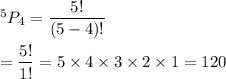
Hence, there are 120 permutations exists of the letters PQRS and T taking four at a time.