Answer:
5
![\sqrt[\\]{3} \\](https://img.qammunity.org/2022/formulas/mathematics/high-school/ryhz2c19o2q6rmh0viw9g3iy4mjg9hquzr.png) or about 8.66
or about 8.66
Explanation:
To find the distance of any line, you have to use distance formula.
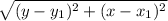
So, we insert our points:
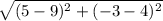
Then you solve, and should end up with
 . Either you can simplify it for an exact number, or you can round. It depends on what the directions ask for.
. Either you can simplify it for an exact number, or you can round. It depends on what the directions ask for.