B) Given
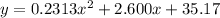
Set y=65 and solve for x, as shown below
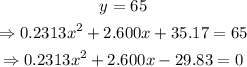
Then, solve the quadratic equation using the quadratic formula,
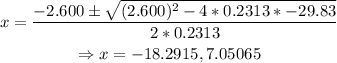
The function is not valid for negative values of x; therefore, the solution can only be x=7.05065 which can be rounded to x=7. Furthermore, x=7 corresponds to the year 2001. The answer to part B is 2001.