Question

Write the equation of a line that is parallel toy= x - 4 and that passes through the point(9,-6). Write your answer in slope-intercept form, y=mx + b.Help please:(

Answer 1

y = x - 15

Explanation:

We have been told to find the equation of a line that is parallel to
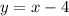`y = x -4` and also passes through the point (9, -6).

In order to find the equation of a line, we need to know its slope and the coordinates of a point that it passes through. We already know of such a point and just need to find the line's slope.

The slopes of two parallel lines are always equal, and therefore if we can find the slope of the line
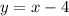`y = x -4`, we will have the slope of our required line as well.

If we compare the equation
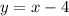`y = x -4` with the general slope-intercept form,
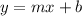`y = mx + b`, we see that m corresponds to 1. Therefore, the slope of
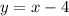`y = x -4` is 1.

This also means that the slope of our required line is 1. Now that we have the slope of the line, we can use the following formula to find the equation of the line:

`\boxed{y - y_1 = m(x - x_1)}`,

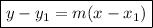where
`(x_1, y_1)` are the coordinates of a point that the line passes through.

Replacing
`m` with 1, and
`(x_1, y_1)` with (9, -6) in the above formula, we get:

`y - (-6) = 1(x - 9)`

⇒
`y + 6 = x - 9`

⇒
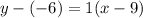
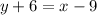
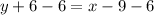`y + 6 - 6= x - 9 - 6` [Subtracting 6 from both sides of the equation]

⇒
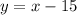`y = x - 15` (Answer)

Therefore the required equation is y = x - 15.