Answer:
A.
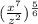
Step by step explanation:
We have been given an radical expression
![(\sqrt[6]{x^7z^(-2)})^5](https://img.qammunity.org/2018/formulas/mathematics/high-school/yy124tsg3e4ljvbrstgjhu64lkd97lurb0.png) and we are asked to choose the correct option which represent our expression with a rational exponent.
and we are asked to choose the correct option which represent our expression with a rational exponent.
Using exponent rule for negative exponents
 we can write our expression as:
we can write our expression as:
![(\sqrt[6]{x^7*(1)/(z^(2))})^5](https://img.qammunity.org/2018/formulas/mathematics/high-school/jtupsdigmwloxry1kj6a6hh92utlohfyf7.png)
![(\sqrt[6]{(x^7*1)/(z^(2))})^5](https://img.qammunity.org/2018/formulas/mathematics/high-school/amd0aw9mncwkduihap5oe1usfvycnlf3mh.png)
![(\sqrt[6]{(x^7)/(z^(2))})^5](https://img.qammunity.org/2018/formulas/mathematics/high-school/f478lvzr2aldmtb2jnxbu3v2wc4g4i8dlp.png)
Using the exponent property
![\sqrt[n]{a}=a^{(1)/(n)}](https://img.qammunity.org/2018/formulas/mathematics/high-school/9afgj7efz54z6g8d616lh2cwxntr528u7e.png) we can write our expression as:
we can write our expression as:
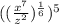
Using exponent property
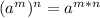 we can write our expression as:
we can write our expression as:
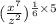
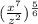
Therefore, after representing our expression with rational exponent our given expression will be:
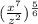 and option a is the correct choice.
and option a is the correct choice.