Answer:
Zero product property is the most appropriate explanation for Line 4
Explanation:
Given the equation:
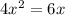
Line 1.
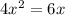 [Given]
[Given]
Line 2.
Addition property of equality states that you add same number to both sides of an equation.
Add -6x to both sides we have;
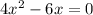
Line 3.
Using the distributive property:
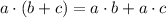
then;

Line 4.
Zero product property states that if xy = 0 then either x = 0 or y = 0
by zero product property we have;
2x = 0 or 2x -3
⇒x = 0 or 2x = 3
⇒x = 0 or x = 3/2
Therefore, the most appropriate explanation for Line 4 is, Zero product property