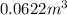Question

A girl is floating in a freshwater lake with her head just above the water. If she weighs 610 N, what is the volume of the submerged part of her body?

Answer 1

The volume of the submerged part of a girl's body in a freshwater lake while floating can be determined using Archimedes' principle, which equates the buoyant force to the weight of the displaced fluid. With her weight being 610 N and the density of freshwater being 1000 kg/m³, the submerged volume is calculated to be 0.062 m³ or 62 liters.

Step-by-step explanation:

To find the volume of the submerged part of the girl's body in freshwater, we can use the principle of buoyancy, often referred to as Archimedes' principle. This principle states that the buoyant force acting on a submerged object is equal to the weight of the liquid displaced by the object. Therefore, if the girl weighs 610 N and is floating in freshwater, the volume of water she displaces while floating must have a weight of 610 N.

Freshwater has a density (ρ) of approximately 1000 kg/m³, and weight can be calculated using the formula weight (W) = mass (m) × gravitational acceleration (g). Since weight is equal to the force of buoyancy in this case, and we know the girl weighs 610 N, we can set up the following formula to find the displaced volume (V):

W = V × ρ × g

610 N = V × 1000 kg/m³ × 9.81 m/s²

Solving for V gives us the volume of the submerged part of her body. V = 610 N / (1000 kg/m³ × 9.81 m/s²) = 0.062 m³ or 62 liters.

Answer 2

The volume of the submerged part of her body is
`0.0622m^(3)`

Step-by-step explanation:

Let's define the buoyant force acting on a submerged object.

In a submerged object acts a buoyant force which can be calculated as :

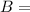`B=`ρ.V.g

Where ''B'' is the buoyant force

Where ''ρ'' is the density of the fluid

Where ''V'' is the submerged volume of the object

Where ''g'' is the acceleration due to gravity

Because the girl is floating we can state that the weight of the girl is equal to the buoyant force.

We can write :

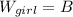`W_(girl)=B` (I)

Where ''W'' is weight

⇒ If we consider ρ =
`1000(kg)/(m^(3))` (water density) and
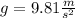`g=9.81(m)/(s^(2))` and replacing this values in the equation (I) ⇒

`B=W_(girl)`

`B=610N`

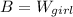
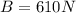
ρ.V.g = 610N

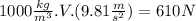`1000(kg)/(m^(3)).V.(9.81(m)/(s^(2)))=610N` (II)

The force unit ''N'' (Newton) is defined as

`N=kg.(m)/(s^(2))`

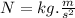
Using this in the equation (II) :

`(9810(N)/(m^(3))).V =610N`

`V=(610N)/(9810(N)/(m^(3)))`

`V=0.0622m^(3)`

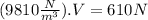
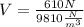
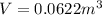
We find that the volume of the submerged part of her body is
`0.0622m^(3)`