Question

How many pounds of nuts worth $1.10 per pound must be mixed with 36 pounds of candy worth $1.60 per pound to produce a party mix worth $1.50 per pound?

a. 9
b. 20
c. 114.5

Answer 1

the answer is 9 pounds.

Answer 2

9 pounds

Explanation:

Let the amount of nuts be x

Cost of 1 pound nuts is $1.10

So, Cost of x pounds = $1.10 x

Cost of 1 pound of candy = $ 1.60

Cost of 36 pounds of candy =
`1.60 * 36=57.6`

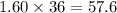
So, total cost = 1.10 x + 57.6

Total weight = x+36

Cost of mixture per pound =
`\frac{\text{total cost}}{\text{total weight}}`

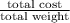
Now we are given that Cost of mixture per pound is $1.50

So,
`(1.1x+57.6)/(x+36)=1.50`

`1.1x+57.6=1.50(x+36)`

`1.1x+57.6=1.50x+54`

`57.6-54=1.50x-1.1x`

`3.6=0.4x`

`(3.6)/(0.4)=x`

`9=x`

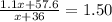
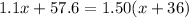
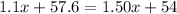
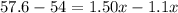
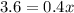
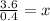
Hence 9 pounds of nuts worth $1.10 per pound must be mixed with 36 pounds of candy worth $1.60 per pound to produce a party mix worth $1.50 per pound.