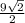Answer:
radical form of the fraction is
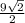
Explanation:
We have to simplify the radical expression by rationalizing the denominator.
Given fraction is

we will simplify the numeration and denominator of the given fraction.
Numerator
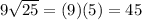
Denominator
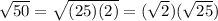
=
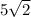
Now the fraction is
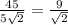
Now for radical expression we will multiply with
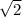 with numerator as well as denominator both
with numerator as well as denominator both
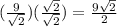
Therefore, radical form of the fraction is