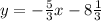Question

Write the equation of the line that is equidistant from the points (-11, -8) and (-1, -2).Write the equation in slope-intercept form.

Answer 1

`y=-(5)/(3)x-8(1)/(3)`

Step-by-step explanation:

A line equidistant from the points (-11, -8) and (-1, -2) will be a line perpendicular to the given line.

Step 1: Find the midpoint of the line

`\begin{gathered} M(x,y)=\mleft((x_1+x_2)/(2),(y_1+y_2)/(2)\mright) \\ =\mleft((-11+(-1))/(2),(-8+(-2))/(2)\mright) \\ =(-(12)/(2),-(10)/(2)) \\ =(-6,-5) \end{gathered}`

Step 2: Find the slope of the line:

`\begin{gathered} \text{Slope},m=\frac{Change\text{ in y-axis}}{Change\text{ in x-axis}} \\ =\frac{-2-(-8)_{}}{-1-(-11)} \\ =(-2+8)/(-1+11) \\ =(6)/(10) \\ m=(3)/(5) \end{gathered}`

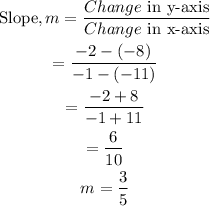
• Two lines are perpendicular if the product of their slopes is -1.

Since the new line is perpendicular, its slope will be:

`n=-(5)/(3)`

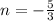
Step 3: Find the equation of the line

We are then to find the equation of a line passing through (-6,-5) with a slope of -5/3.

Using the point-slope form:

`y-y_1=m(x-x_1)`

We have:

`\begin{gathered} y-(-5)=-(5)/(3)(x-(-2)) \\ y+5=-(5)/(3)(x+2) \\ y=-(5)/(3)(x+2)-5 \\ y=-(5)/(3)x-(10)/(3)-5 \\ y=-(5)/(3)x-8(1)/(3) \end{gathered}`

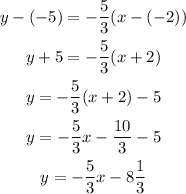
The equation of the line equidistant from the points (given in slope-intercept form) is:

`y=-(5)/(3)x-8(1)/(3)`