STEP - BY - STEP EXPLANATION
What to find?
Determine whether the given series converge or diverge.
Given:
Step 1
Determine the common ratio.
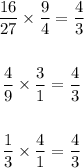
It is enough to see that it is a geometric series.
Step 2
List out the conditions for convergence /divergence of a geometric series.
• If the absolute value of the ,common ration, i.e, |r| is less than 1,, the the series ,converges,.
,
• If, |r| > 1, then the series, diverges.
Clearly, 4/3 > 1
This implies |r| >1, hence the series diverges.
ANSWER
The series diverges.
The series is geometric and the absolute value of the common ratio is greater than 1.