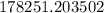Answer:
Explanation:
We have given:
Principal amount which is 80,000
Time which is 20 year
Rate which is 11.5%
And since, we have to find 13 years early so, time would be: 20-13=7 years.
And since, we have to find for 12 months
Hence, n=12
We have formula to calculate compound interest:
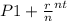
On substituting the values we get:
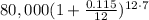
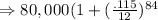
On simplification we get: